Numbers are captivating, aren’t they? Whether we recognize or no longer recognize it, it forms how we assume, work, and remedy problems. Today, we’re diving into a selected sequence you’ve seen in a few instances: 30 40 50 40 45 50. It seems like a random string of numbers in the beginning look; however, there’s more to it than meets the eye.
This isn’t just any set of numbers—it can train us in something treasured, such as mathematics patterns, intellectual math, and real-world applications. If you’re prepared to crack the code, permits get commenced!
Understanding the Sequence: 30, 40, 50, 40, 45, 50
At first, the numbers 30, 40, 50, forty, 45, and 50 would look like they’re leaping all over the vicinity. But as with most matters in math, there’s a hidden structure that is famous itself when you look a bit nearer.

So, what’s the sample right here? If you destroy it down grade by grade, you’ll notice that the series involves both additions and subtractions:
- Thirty to forty is a boom of 10.
- Forty to 50 is also a growth of 10.
- But then, from 50 to 40, there’s a decrease of 10.
- After that, forty to forty-five goes up by using five.
- And in the end, 45 to 50 will increase with the aid of five once more.
The first part of the sequence follows a pattern of including 10, then subtracting 10. The second part reduces the step size to 5, increasing and ending with any other jump to 50.
The Arithmetic Secrets Behind the Sequence
Now that we’ve laid out the sequence let’s break down the arithmetic secrets and techniques hidden within the numbers. The adjustments between 30 40 50 40 45 50 may also appear simple at the start. However, they tell a story of ways numbers interact with each other and, extra importantly, how we can manage them in numerous approaches.
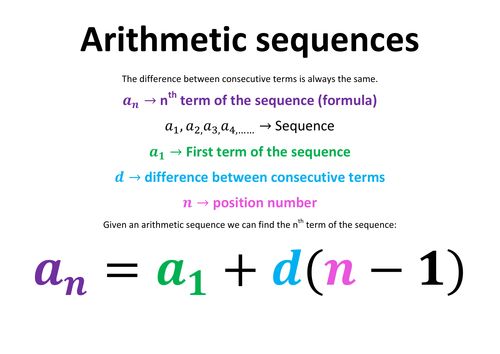
Identifying the Pattern
The beauty of this collection lies in its mixture of mathematics development and alternating patterns. The first set (30, 40, 50) paperwork a clean arithmetic progression, wherein every variety is ten devices apart. This is an honest example of how sequences work in simple mathematics. A mathematics development like this occurs when you continually add an equal fee.
But then, the sample shifts. The transition from 50 to 40 breaks the pattern of continuous addition. Here, in preference to following via with some other 10-point jump, the series decreases by using 10, giving us a glimpse of ways styles can exchange and become more complex.
The collection’s second half (forty, 45, 50) introduces a more minor step: Cost. The development shrinks to a difference of 5, increasing and decreasing. This trade highlights how numbers can transition between one-of-a-kind scales simultaneously while maintaining a predictable flow. These fluctuations from 10 to five show how arithmetic patterns can be extra nuanced.
Practical Applications of the Sequence
Understanding sequences like 30, 40, 50, 40, 45, and 50 goes beyond just numbers on a page. These types of styles are the building blocks of real-international eventualities. Whether you’re calculating boom prices, predicting tendencies, or studying record sets, spotting these progressions is critical.

For example, in economic analysis, sequences like this can constitute fluctuating market tendencies, in which boom and decline occur in varying increments. Apprehending those styles enables traders to make extra informed choices by looking ahead to the subsequent movement in a series.
The pattern of 30, 4050, 40, 45, and 50 also mirrors the adjustments we see in regular situations, like temperature shifts over the years or adjustments in stock levels in a business setting. Knowing how to spot and expect these styles can enhance selection-making and forecasting.
Case Study: Real-World Application of Arithmetic Sequences
Let’s briefly dive into a case examination regarding sequences like 30, 40, 50, 40, forty-five, and 50.

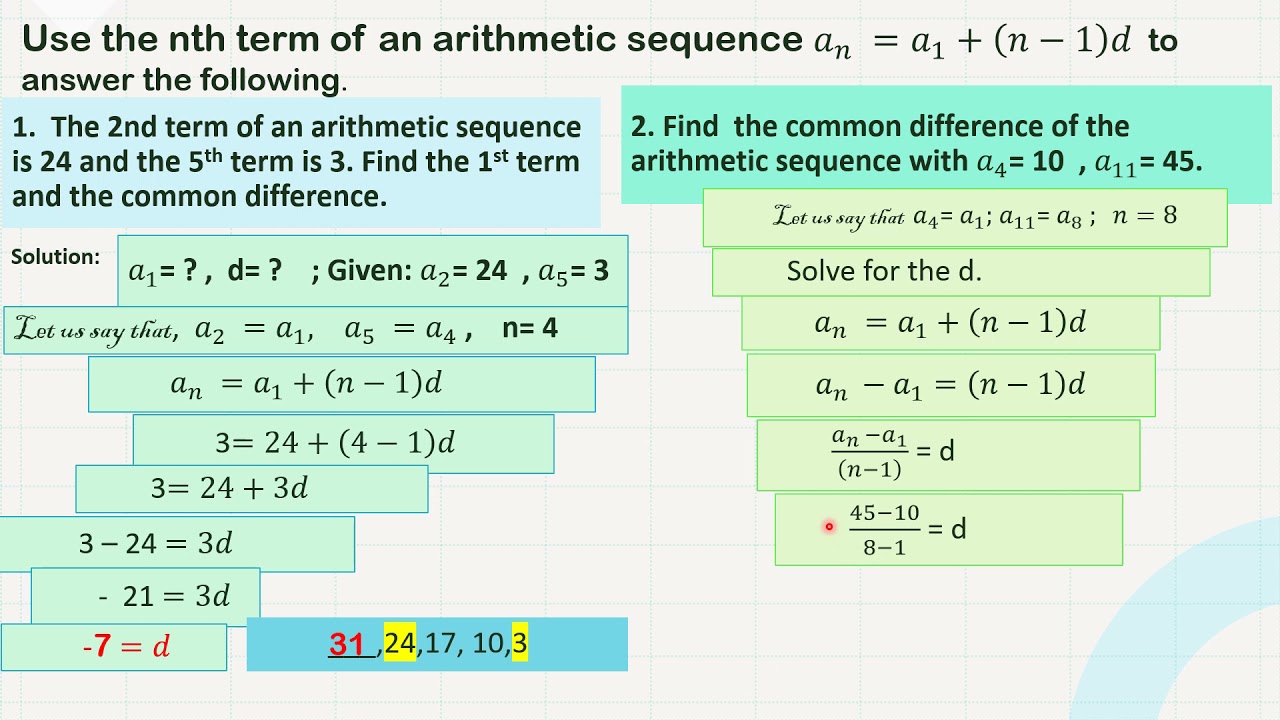
Imagine you’re managing a task with fluctuating weekly budgets. In week 1, you’ve got $30,000 available. By week 2, your budget will increase to $40,000; in week three, it’s up to $50,000. But in week four, you experience a reduction, losing the finances to $forty 000. There’s a sluggish healing in weeks five and six, with $45,000 and $50,000, respectively.
The capability to apprehend this sequence permits you to anticipate changes in future weeks. By knowing the pattern, you may allocate assets more efficiently and plan for an increase or decrease in funding. This type of evaluation can prevent overspending or underspending and ensure the easy execution of the project.
Applying This Concept in Education
Teachers use these sequences to help college students grasp the idea of trade over time. Introducing styles like 30, 4050, 40, forty-five, and 50 right into a schoolroom setting can illustrate the fundamentals of mathematics sequences. This not only aids in studying basic math principles but also facilitates college students’ increasing crucial thinking abilities by identifying developments and fixing issues more efficiently.
[ruby_related heading=”More Read” total=5 layout=1 offset=5]
1. What is the mathematics series in this pattern?
The mathematics series in 30, 40, 50, 40, forty-five, and 50 takes place in parts. The first component (30, 40, 50) entails a step length of 10, even as the second component (40, forty-five, 50) entails a step length 5.
2. How can I observe this collection in actual existence?
This sample may occur when numbers boom and decrease in regular steps, including budgeting, forecasting, and fashion evaluation.
3. Why does the step size trade within the center of the sequence?
The trade-in step length from 10 to five illustrates how styles can shift over time, mimicking actual-world situations where the pace of trade isn’t constantly steady.
4. Is this a typical mathematics pattern?
While combining growing and reducing steps with various sizes isn’t the most common mathematics pattern, it’s commonplace. Sequences like those are frequently visible in alternating trends or irregular progressions.
5. Can I expand this collection further?
Absolutely! You can predict future numbers by following the alternating pattern of 10s and 5s. After 50, you could anticipate a decrease back to 45, observed through any other leap to fifty-five, persevering with the progression.
Why Understanding Arithmetic Sequences Matters
So, what’s the takeaway from all this? Recognizing and having expertise in mathematics styles, like the one in 30, forty 50, 40, forty-five, and 50, isn’t fixed math issues. It’s about growing a mindset that helps you approach complicated conditions in a logical, structured way.
In a world driven by facts, these patterns shape the muse of the whole lot, from financial forecasting to medical evaluation. The capacity to interrupt down and apprehend the numbers offers you a side, whether you’re handling finances, predicting income, or seeking to make feel of normal fluctuations.
Once you start looking for it, you’ll see these arithmetic styles everywhere—from how matters develop to how they decline, get better, and develop once more. With a touch exercise, you could master the art of spotting and using them for your gain. And that’s where the proper electricity of arithmetic lies.
So next time you see a series like 30, 40, 50, 40, 45, or 50, don’t just pass it off as random. Dive in, appearance closer, and spot what secrets the numbers have to show!